Spherical Near-Field Measurements
The spherical near-field measurement is a method for measurement of the radiation pattern of an antenna. The advantage compared to far-field measurement ranges is the reduced distance between the AUT and probe/feed, since the probe does not have to be in the far-field of the AUT. However, a transformation of the near-field data is necessary to obtain the far-field pattern. The transformation method is based on the Huygens principle: From the measured tangential near-field components on a defined area (here: sphere), the far field is determined by calculation.
Transformation Algorithm
The standard solution method is based on Fourier transforms. This approach is very quick and efficient. However, the data must be measured on an equidistant grid and a probe correction is exact only for certain types of probes (µ=±1). Therefore, new transformation algorithms are investigated at the IHF which do not have these limitations. The activity focuses mainly on methods which require solving a linear equation system. Additionally, methods are explored which for example calculate the currents on the antenna aperture in order to detect errors. These methods can be used for antenna analysis.
Accelerated Test Methods
In spherical near-field measurements field data is always needed on a closed sphere around the AUT. This leads to a large number of measurement points and a long measuring time. For studies of individual antenna parameters (eg HPBW) or a throughput of antennas the measurement time is a critical factor. Therefore, various methods of acceleration are investigated at the IHF. This involves checking, for example, which areas need to be measured in the near-field to achieve a certain accuracy in the far-field. Correspondingly what methods are suitable for extrapolation. This reduction of the measurement points (truncation) shortens the measurement time significantly. Current work investigates how single pattern cuts can be transformed to the far-field. For certain antennas it is even possible to calculate the far-field from single cut near-field measurements. These methods have the drawback that the far-field is only approximated.
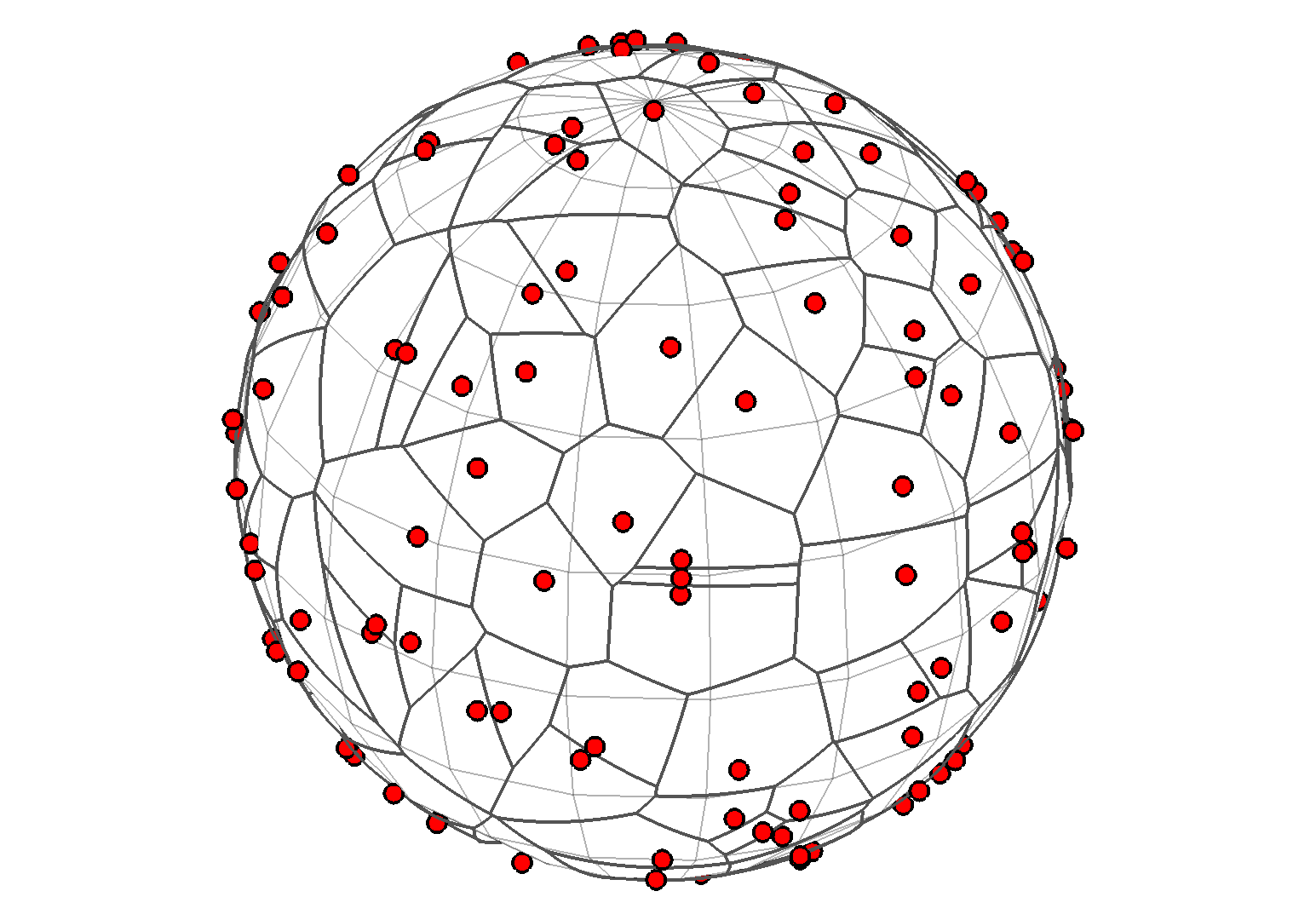
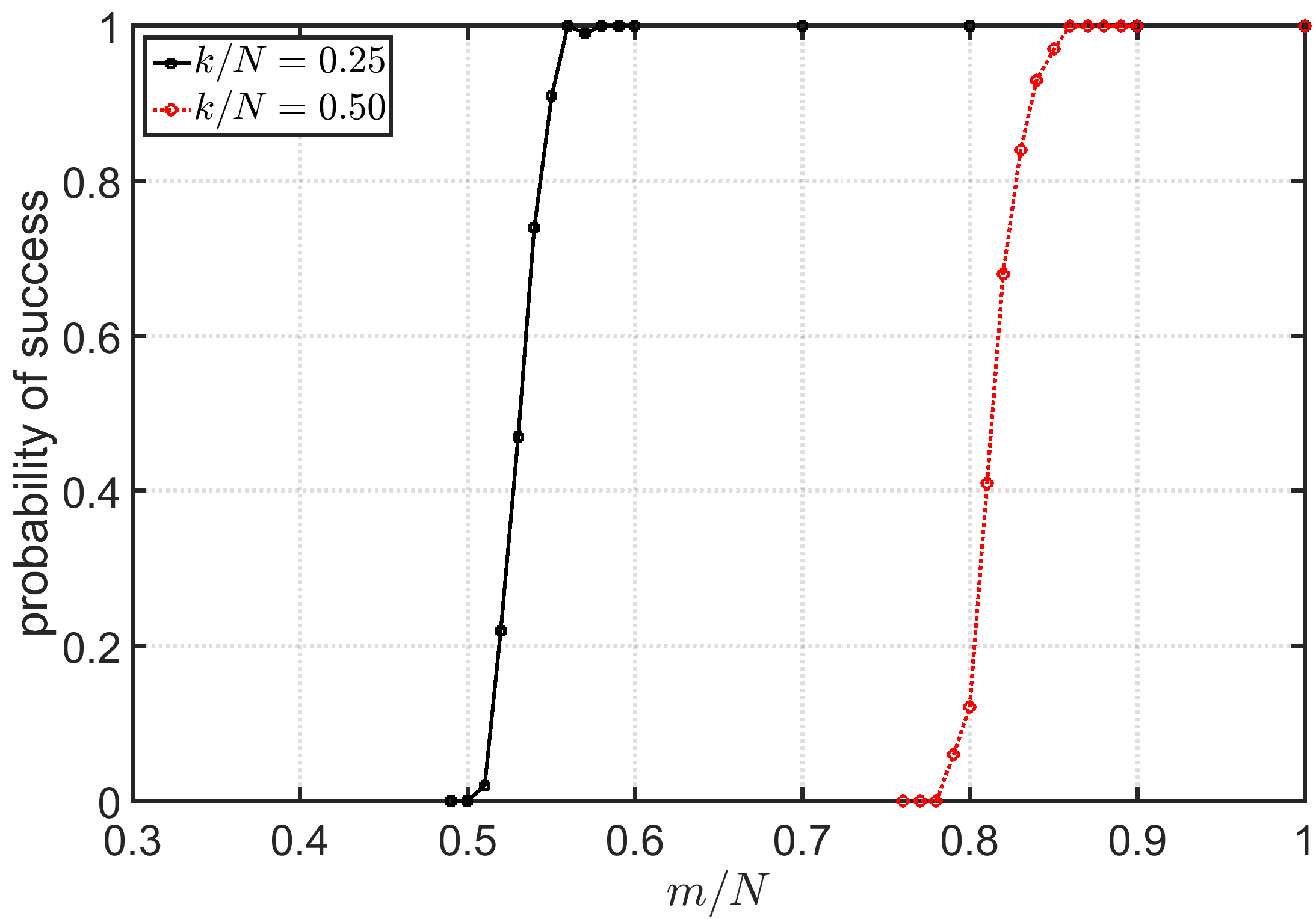
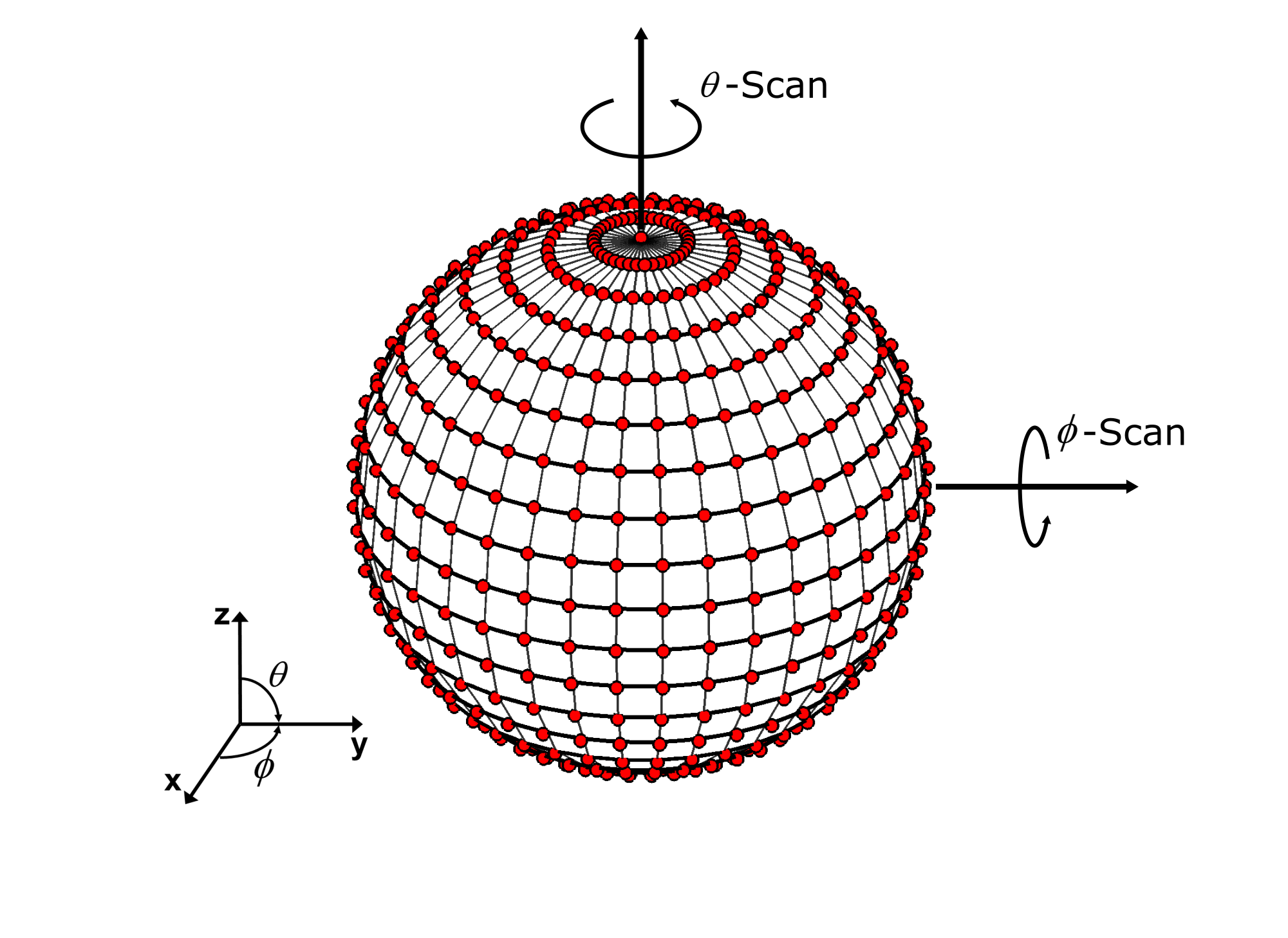
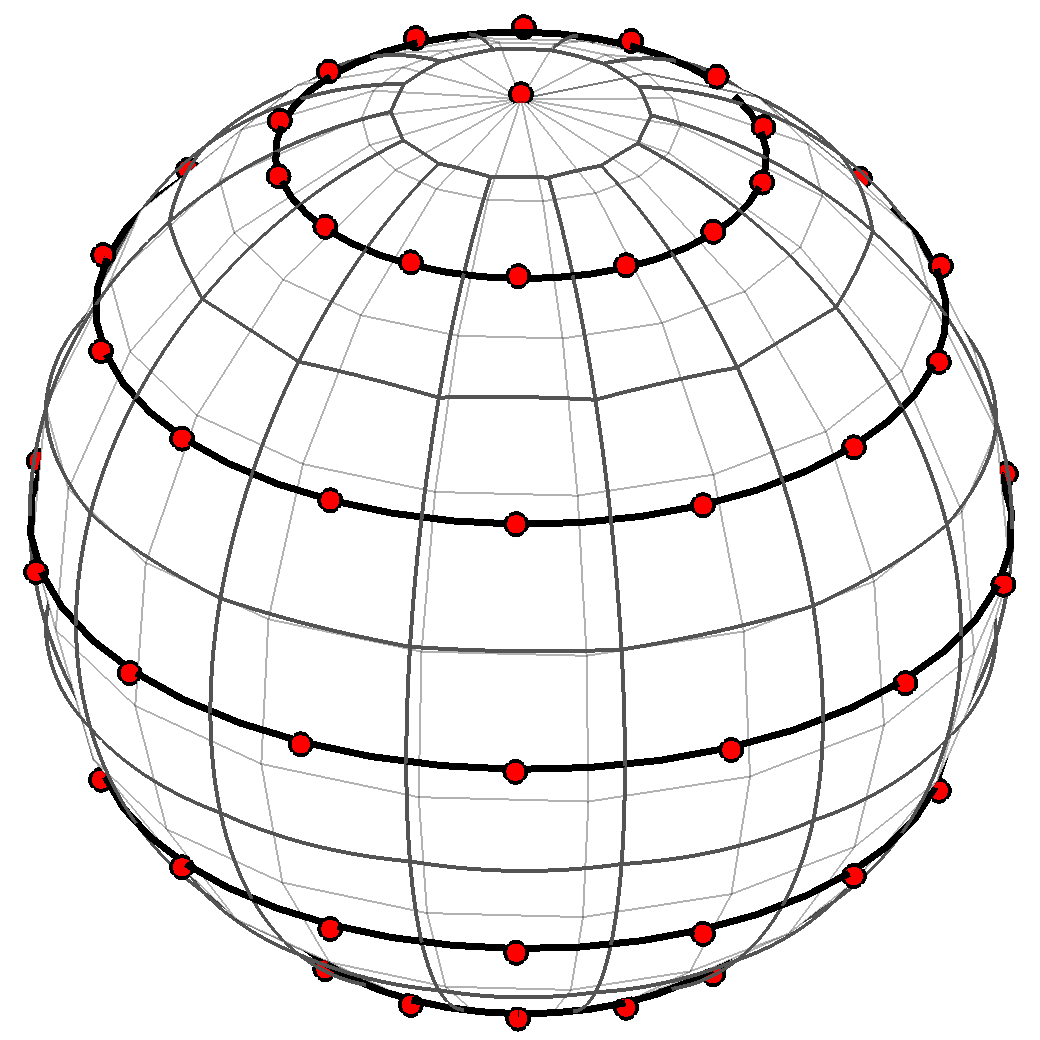
To overcome this limitation methods are investigated to reduce the number of measurements without loss of accuracy. Due to the typical regular spherical sampling the measurement point density at the pole is very high. The linear equation system is therefore highly overdetermined. Solving linear equation system instead of using Fourier transformations allows arbitrary sampling schemes which were investigated. Figure 1 and 2 show as example the regular and a spiral sampling grid.
In a next step we will investigate how compressed sensing methods for underdetermined systems can be exploited in the spherical near-field to far-field transformation.
Compressed Sensing for Spherical Near- to Far-Field Transformation (CoSSTra)
Supported by the German Research Foundation
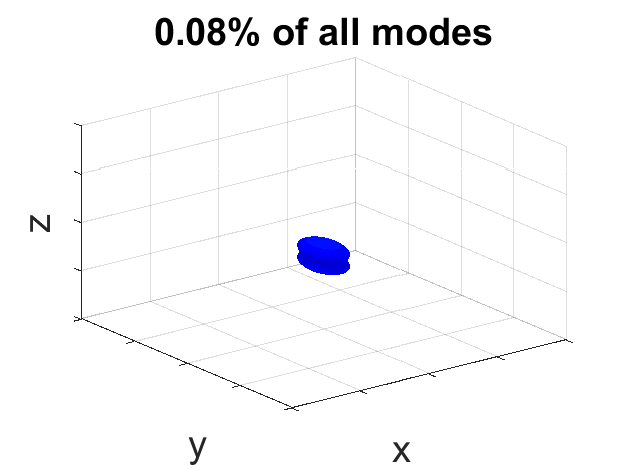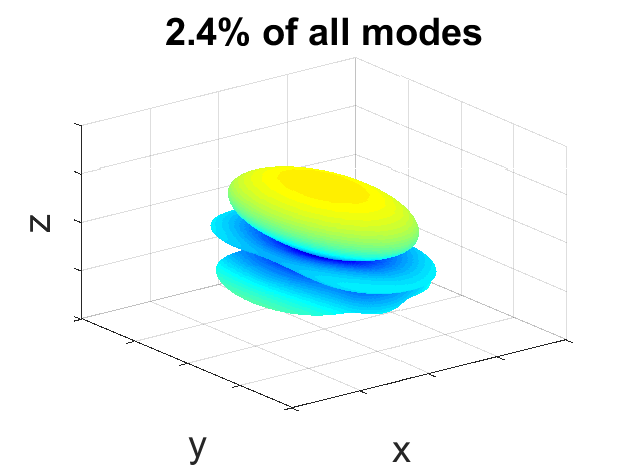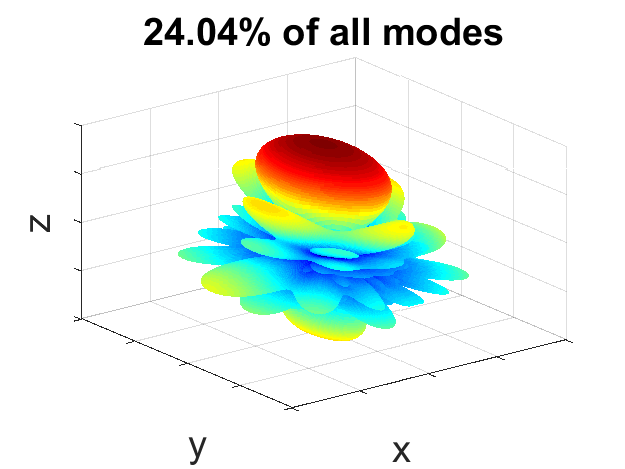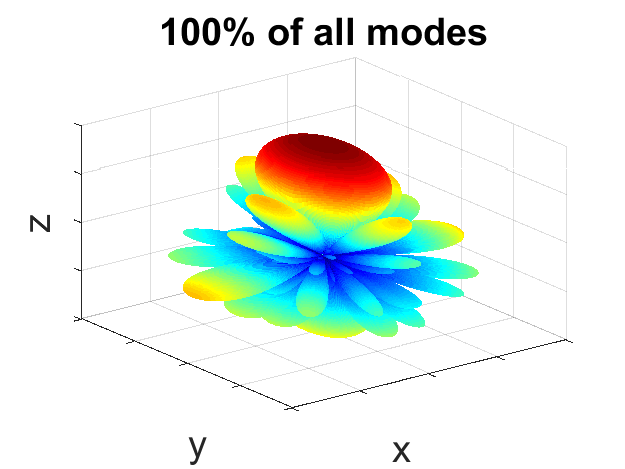
Due to diverse and increasing demands for antennas regarding bandwidth (e.g. for ultra-wideband antennas), radiation properties (e.g. for reconfigurable antennas) and high operating frequencies (e.g. for radar systems in the automobile sector), the complexity of antennas as well as their measurement efforts has increased greatly in recent years. In order to save time, often only partial measurements with a small selection of frequencies and antenna configurations are carried out. Particularly in the near-field, the long measurement period is a strong limiting factor. The reason for this is the high number of measurement points that have to be acquired in order to determine the transmission characteristics of an antenna with satisfactory accuracy. However, if the radiation of the antenna is described in terms of spherical waves, it turns out that in practice the relevant information is mostly concentrated in a few spherical wave coefficient.
The project aims to bring together methods of compressed sensing with the theory of spherical near- to far-field transformation to enable subsampled antenna measurements on the sphere with minimal loss of accuracy. To this end, different approaches for signal reconstruction are initially considered to integrate structural properties of the spherical mode spectrum in existing solutions. Methods are developed that, based on prior knowledge about the antenna characteristics, provide information about selecting an optimal basis and restrict the search space of optimal solutions. For a reliable reconstruction of the subsampled signal, it is also necessary to select the required number of measurement points appropriately. For this purpose, it is studied which antenna characteristics lead to sparse mode spectra. Another part of the project aims at identifying appropriate sampling strategies on the sphere, enabling a robust reconstruction of the spherical modes. In this context, the as yet unexplored influence of the polarization angle on the reconstruction behavior is determined. An important part of the project aims at the identification of measurement uncertainties when using the new methods, which are determined by extensive analysis. An interesting method in this context is the so-called Bayesian Compressed Sensing which allows for obtaining confidence intervals for the calculated solutions during reconstruction. In the final step, the explored approaches will be verified on the basis of extensive measurements of various types of antennas.
The research results should answer the question how compressed sensing can be used in the context of spherical near- to far-field transformation for subsampling of antenna measurements.